https://doi.org/10.1140/epjs/s11734-022-00488-8
Regular Article
Hopf bifurcation and its control in a 3D autonomous system
1
Department of Mathematics, Nanjing University of Aeronautics and Astronautics, 210016, Nanjing, Jiangsu, China
2
Key Laboratory of Mathematical Modelling and High Performance Computing of Air Vehicles(NUAA), MIIT, 210016, Nanjing, Jiangsu, China
Received:
29
November
2021
Accepted:
17
February
2022
Published online:
1
March
2022
The first section of this paper discusses the stability and Hopf bifurcation for a new dynamical system using stability theory, the center manifold as well as normal form theory. To verify the analytical results, numerical simulations are performed. The second section focuses on controlling the Hopf bifurcation with a robust controller capable of handling a wide range of parameter values. By fine tuning the control parameters, the controller ensures that Hopf bifurcation occurred at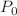 
. Furthermore, we postpone the Hopf bifurcation at 
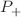by adjusting the control parameters.
© The Author(s), under exclusive licence to EDP Sciences, Springer-Verlag GmbH Germany, part of Springer Nature 2022
