https://doi.org/10.1140/epjs/s11734-023-01035-9
Regular Article
Orbital maneuvers for a space probe around Titania
1
Grupo de Dinâmica Orbital & Planetologia, São Paulo State University (Unesp), School of Engineering and Sciences, Guaratinguetá, CEP 12516-410, São Paulo, Brazil
2
National Institute for Space Research, Av. dos Astronautas, 1758, São José dos Campos, SP, Brazil
3
Academy of Engineering, RUDN University, Miklukho-Maklaya street 6, 117198, Moscow, Russia
Received:
17
May
2023
Accepted:
9
November
2023
Published online:
7
December
2023
For most space missions, it is interesting that the probe remains for a considerable time around the mission target. The longer the lifetime of a mission, the greater are the chances of collecting information about the orbited body. In this work, we present orbital maneuvers that aim to show how to avoid a collision of a space probe with the surface of Titania. Through an expansion of the gravitational potential to the second order, the asymmetry of the gravitational field due to the coefficient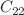 
of Titania, the zonal coefficient 
, and the gravitational perturbation of Uranus are considered. Two models of coplanar bi-impulse maneuvers are presented. The first maneuver consists of transferring an initial elliptical orbit to a final circular orbit, and the second has the objective of transferring an initial elliptical orbit to a final orbit that is also elliptical. The lag in the inclination and semi-major axis of the orbits is investigated before performing the maneuvers. To point out the best scenarios for carrying out the maneuvers, a study is presented for different points of an orbit where transfers could be made. In addition, a maneuver strategy is presented to correct the variation of the periapsis argument. The results show that maneuvers performed a few days after integration are more economical than maneuvers performed later, a few days before the collision. The economy of the maneuvers is also demonstrated through an analysis of the ratio of the increase in speed to the lifetime.
Antônio Bertachini A. Prado, Silvia Giuliatti Winter and Andre Amarante have contributed equally to this work.
Copyright comment Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
© The Author(s), under exclusive licence to EDP Sciences, Springer-Verlag GmbH Germany, part of Springer Nature 2023. Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
