https://doi.org/10.1140/epjs/s11734-023-00839-z
Review
Instances of higher geometry in field theory
Division of Theoretical Physics, Rudjer Bošković Institute, Bijenička 54, 10000, Zagreb, Croatia
a
athanasios.chatzistavrakidis@irb.hr
Received:
15
July
2022
Accepted:
14
April
2023
Published online:
5
May
2023
Generalisations of geometry have emerged in various forms in the study of field theory and quantization. This mini-review focuses on the role of higher geometry in three selected physical applications. After motivating and describing some basic aspects of algebroid structures on bundles and (differential graded) Q-manifolds, we briefly discuss their relation to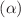 
the Batalin–Vilkovisky quantization of topological sigma models, 
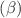higher gauge theories and generalized global symmetries and 
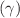tensor gauge theories, where the universality of their form and properties in terms of graded geometry is highlighted.
S.I.: Noncommutativity and Physics. Guest editors: George Zoupanos, Konstantinos Anagnostopoulos, Peter Schupp.
Copyright comment Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
© The Author(s), under exclusive licence to EDP Sciences, Springer-Verlag GmbH Germany, part of Springer Nature 2023. Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
